Main threads in the research work

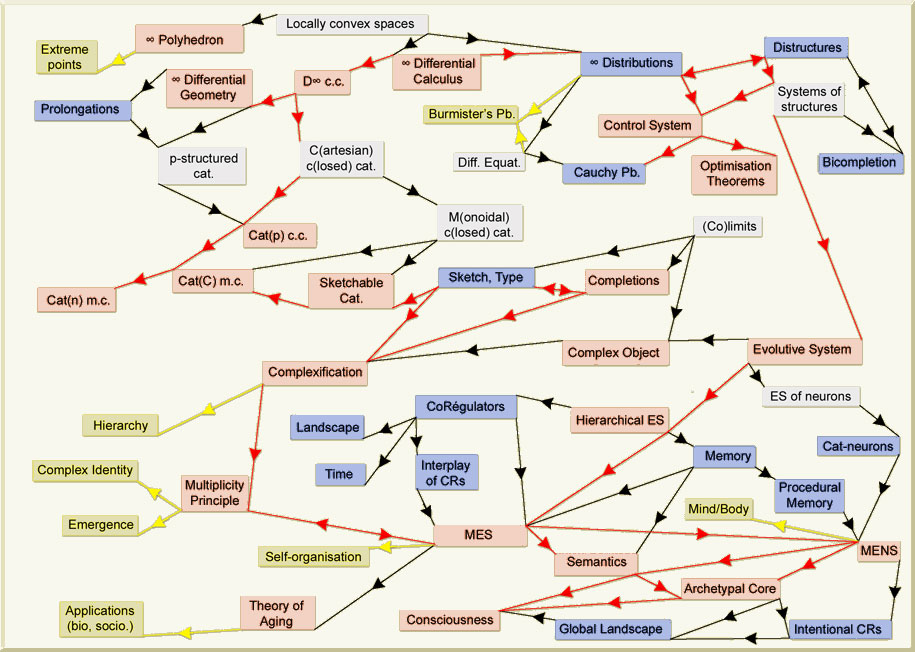
In this graph, the white nodes represent domains to which my research is related, the red and blue nodes the notions I have developed; the links in red indicate the main connections between them.

The first part (from 1957 to 1967) can be classified as analysis (even if categorical notions are introduced in their treatment), those in the second part (from 1968 to 1979) as 'pure' category theory, and the third part concerns applications of the preceding parts to a categorical model for biological and cognitive systems.

This diagram makes explicit three interrelated main threads, all deriving from the initial problem that is raised in my thesis: to unify the various notions of 'generalized functions' and adapt them to the infinite dimensional case, the basic idea under the categorical notion of 'distructure' thus obtained being to extend the differential operators to continuous functions.

1. Infinite dimensional differential calculus and differential geometry, later leading to the study of internal categories and cartesian closed structures.

2. Enrichment and (pre)sheafification of partial category actions (systems of structures in the later terminology of C. Ehresmann), leading to the theory of distructures generalizing Schwartz distributions; their application to model and solve control problems (Control Systems) and, later, to model living systems (Memory Evolutive Systems, with J.-P. Vanbremeersch).

3. Completion problems: bicompletion for distructures, theory associated to a sketch, cartesian and monoidal closed structures on sketchable categories (in particular on the category of multiple categories), complexification process to model the development of Memory Evolutive Systems and the formation of higher cognitive processes up to consciousness for cognitive systems.