WHY?

The Memory Evolutive Systems (MES) propose a mathematical model for autonomous evolutionary systems, such as 'living' systems. This model, based on a particular domain of Mathematics, Category Theory, provides a framework to study and possibly simulate the structure of these systems and their dynamic behavior.

Which is the interest to design models? Since their beginnings, the dream of philosophy and science has been to give an explanatory account of the Universe, to understand what is life, consciousness,.... But as our depth of knowledge progresses, the more it reveals the complexity of what remains to be studied, requiring increasingly elaborate mathematical tools. We can hope that a model brings some light on what characterizes a complex evolutionary system, on what distinguishes it from inanimate physical systems, on its functioning and its evolution in time, from its birth to its death.

Moreover, the behavior of such a system depends heavily on its former experiences which it can memorize for later uses. A model representing the system over a certain period, with its responses to the various situations which it has encountered, could anticipate its later behavior, and perhaps even predict some evolutionary alternatives. This dream of the forecast, like finding a Pythia of modern times, was considerably stimulated by the increasing power of computers, which makes it possible to treat a large amount of data, be they numerical or not, quickly and effectively; but computation also has its limits.

The role of a model will be thus two-fold: theoretical for a comprehension of a fundamental nature, practical for applications in biology, medicine, sociology, ecology, economy, meteorology, etc...

There are many ways of designing models. The traditional models in Physics (inspired by the Newtonian paradigm) generally use a representation based on observable parameters which satisfy systems of differential equations translating the laws of Physics, possibly with chaotic behavior. Such models are often imported in Biology and Ecology. The parameters (real numbers or vectors) are given in an empirical way, starting from experimental observations. These analytical models took even more importance with the development of powerful data processing allowing to handle systems of equations with many parameters.

The 'black box' models do not try to reproduce the internal behavior of a system; they take only into account the inputs, the outputs and the change of state rules. These rules are often of a more formal nature, as in the Turing machines or in the cellular automata of the initiator of the modern digital computer, von Neumann. They can lead to decisional trees which operate on variables issued from data bases according to usual Boolean logic, if... and/or..... then... if not..... etc.... Such trees are used in expert systems, for example for the diagnosis and the treatment of diseases, or factories scheduling.

Cybernetics was defined by Wiener in 1947 as " the entire field of control and communication theory, whether in the machine or in the animal ". Its models use in an essential way Shannon's information theory and the concept of 'feedback'. It developed during the forties, fifties and sixties, thanks to the collaboration of specialists in biology, neurobiology and economy who realized that by comparing their specific approaches, they found a number of analogies in the structure and the evolutionary modes of the systems they studied.

It is also in this multidisciplinarity that Systems Theory developed. It is closely related to Cybernetics, though it focuses more on the structure of systems and on their models than on how they control their actions. The systemic approach is distinguished from the analytical approach by the particular emphasis it lays on the relations of any nature between the components of a system. As defined by von Bertalanffy as soon as 1926, a system is a set of elements with interactions between them organized according to a goal.

Cybernetics and Systems theory are now integrated in the recent 'Science of complexity', which includes the most various approaches: Artificial intelligence, neural systems, catastrophe theory (Thom), chaos, fractals (Mandelbrojt), autopoietic systems (Maturana and Varela), anticipatory systems (Rosen),...

Most of these models lead to various simulations, for example for pattern recognition, weather prediction or financial analysis. Their results are valid locally, e.g. they give reliable forecasts on the short term. But they are limited to a specific level of complexity and energy, because each level has its own laws; for example the molecular level does not have the same laws as the cell level. As a general rule, it is impossible to extrapolate the comportment of the whole from local features, to understand the entire system starting from its parts taken one by one. The more so as each part has its own temporality, and these temporal variations play an essential part in the evolution of a system.

It is no more possible to unify quantum physics and relativity theory than to understand the functioning of the mind from the finest analyses of individual neurons; or to understand how the evolution could lead from some initial macromolecules mingled in a primitive soup to organisms able to develop through exchanges with their environment, to reproduce, to learn and to adapt to changing conditions.

Thus the problem is philosophical. The approach cannot be purely reductionist, but it cannot do without some reductionism. The interactions of some objects, of some building blocks are well understood. The interactions of a very great number of these blocks are actually at the root of their spatial, energetic and temporal organization, but the existence of this organization can only be noted as a fact, to be considered in itself as a new object of a higher level. And it is then necessary to analyze this object in the context of the higher level to possibly understand its functioning.

This is why many authors have introduced a concept of hierarchical systems in the most various fields, for example: in Physics (Reeves, Ullmo), in Biology (von Bertalanffy, Jacob, Monod), in Neurology (Changeux, Jeannerod, Laborit), in Evolution Theory (Dobzhanski, Mayr, Teilhard de Chardin), in Ethology (Lorenz, Tinbergen), in the Social sciences (Koestler, Morin, Piaget). For instance Jacob writes: " Any object which Biology considers represents a system of systems; itself element of a system of a higher nature, it obeys some rules which cannot be deduced from its own analysis. " But it is the passage from a lower level to a higher level which remains obscure in these models; as Farre says (in the preface to Schempp's book): " computational strategies are successively exploited only in the simulation of interactions with a single energetic level of observation ". Indeed, this passage is characterized by what is called emergent properties. To simplify, a brick heap is nothing else than the sum of all its bricks, with some random spaces between them; it is thus characterized by its weight, which is the sum of the weights of the bricks, its volume which is the sum of the volumes of the bricks and of the spaces between them, its more or less arbitrary form. A wall or a dam may correspond to the same bricks, but connected by specific links between themselves and with respect to an object external to them, for example a river. Each brick participates in the object wall or dam in collaboration with the other bricks, and all operate in a 'concerted' way with respect to the river, so that there emerges a global operation of the wall or the dam on the river.

1. In the case of the nervous system, usual models, in particular the connectionist neural systems (following Hopfield), give rather reliable results for carrying out a local analysis: starting from a small number of neurons and some basic rules, it will be possible to obtain an exact simulation of the operation of these neurons on a short enough period. But the problem becomes intractable if we try to extend the model to study the global operation of the brain. Even the description of only one great brain function, for example visual recognition, cannot be reduced to a sum of local analyses. It would be as if we tried to understand the operation of a social group by the sum of the families which belong to it.

The organization of such a function is complex, with a structure which can be described as hierarchical, from the multiple neurons composing the retinal image in the optic nerve to neuronal groups in higher areas: neuronal groups of the visual areas operating a more or less parallel analysis of the various features of an observed object, neuronal groups of the memory activated by these visual areas and implicated in the formation and recognition of the image of the object, neuronal groups of the associative areas integrating the meaning of the perceived image in a semantic memory. In short, there is a process which at each stage becomes more complex by the association of a growing number of interacting neurons activated in a synchronous way; it rests on the rules of cellular neurophysiology (involving observables such as the spiking frequencies of neurons, the transmission delays, the synaptic strengths), and on the relationships of the neuronal groups between them and with other areas. An assembly of various neuronal groups can possibly behave like a new object, confronted then with other data provided by the lower areas. The recognition of an object as being a bottle is not enough to recognize the liquid which it contains, and afterwards, if it is a wine, its nature, its origin, etc...

It is clear that the observables, in particular the time scales, depend in an essential way on the hierarchical level (neurons, groups of neurons, cerebral areas, associative cortex): the duration of a step in the activity of a neuron coming from the retina (i.e. the period encompassing the reception of the signal, its transmission along the axon and through the synapse, the consecutive refractory period) will not be of the same quantitative and qualitative order as the mechanisms concerned in the recognition of a face by a higher area.

Let us give a clinical example, probably a kind of epileptic process, which illustrates this temporal variability. It is characterized by a feeling of acceleration, each gesture, each thought, each action seeming to be conveyed at a speed record, like in a speeded-up film. However, in spite of the disturbing feeling given by this phenomenon, the subject can attempt to analyze the real rhythm of things, as if he were looking from outside at his 'accelerated self'. All occurs then as if he had two levels of consciousness: a first level extremely and abnormally fast, and a second one normal. We could analyze this phenomenon as the unusual irruption at the conscious level of a multitude of fragments of actions or thoughts. This fragmentation is normally unconscious and is reflected at the conscious level only after it is united in a continuous process; but in this case, it becomes conscious as such, each fragment being at the origin of a discontinuity, of a rupture. Instead of a continuous flow, at the rhythm of the thoughts and general activities, this phenomenon introduces a split flow, with decomposition of each complex movement in its successive components, of each thought in its ends of sentence or even words, put end to end.

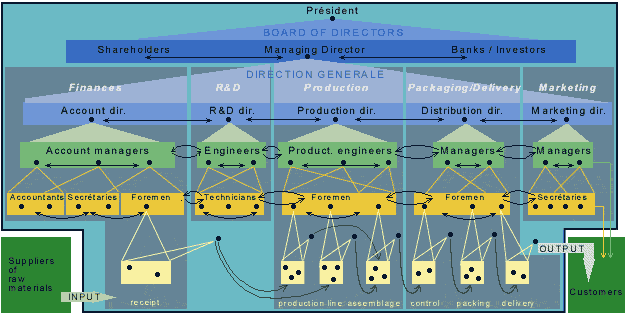
2. Another example is given by a company. It includes several groups of workmen, with well defined functions, using specific tools and raw materials. Their production may be passed on to semi-skilled workers who will assemble the parts initially manufactured. Others will be in charge of controls, others of packaging, of forwarding, etc. These multiple groups will be supervised by foremen, themselves working in collaboration, and under the orders of a higher level managerial staff. The role of this staff thus consists in supervising a whole hierarchy of more or less specialized personnel. This supervisory staff has itself a direction formed by the directors of various departments, who report to the board of directors, and finally to the president of the company. It is clear that the workmen at the end of the chain are not in relation with the same objects, the same tools, the same people, internal or external to the company as the members of the Board of directors. They do not have the same decisional delays, the same rhythm of development of a strategy and of the ensuing actions as the executives. The choice between possible strategies in front of a situation is less broad at the level of the workman, his strategies are less complex, as well in terms of the parameters which it encompasses as of the number of stages in which it will be applied. The selection of a strategy at the higher level, for example to imagine a new production, to search for possible markets, for distribution circuits, for financial arrangements is much more complex and has longer-term repercussions. It will be reflected only later on at the lower levels, the rhythms of decision and effection accelerating gradually from top to bottom.

In the same way, a problem in the supply of raw materials, immediately perceived at the level of the workmen in their daily labor, will require only with delay a strategy of replacement, decided by the higher levels, after analysis of the causes, of the possible consequences, and research of the optimum strategy for a foreseeable future. In this case also, the duration of the stages is very variable, as their quality, for example with respect to the frequencies of activation, the number and the complexity of the parameters to be analyzed, the number and the complexity of the choices of strategy to be applied.